
You use the opposite number because multiplication and division are also opposites. However, when you divide by a fraction you flip the fraction over so the numerator is on the bottom and the denominator is on top.
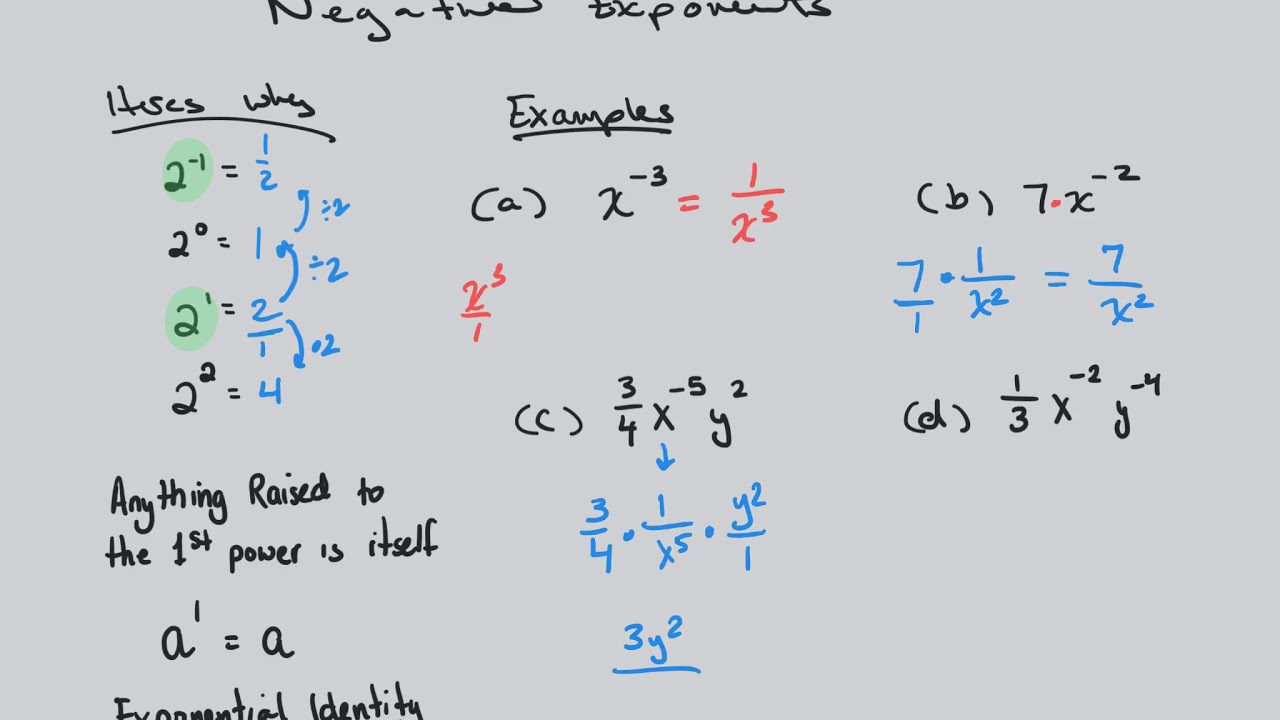
The numerators get multiplied, and the denominators get multiplied. (If not, you can always check out our lesson on multiplying and dividing fractions.) When you multiply two fractions, you multiply straight across. If you've ever multiplied and divided fractions, the reciprocal might seem familiar to you.
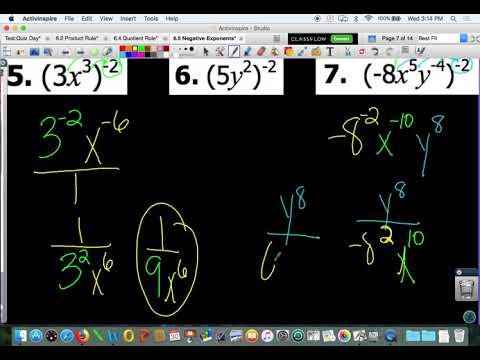
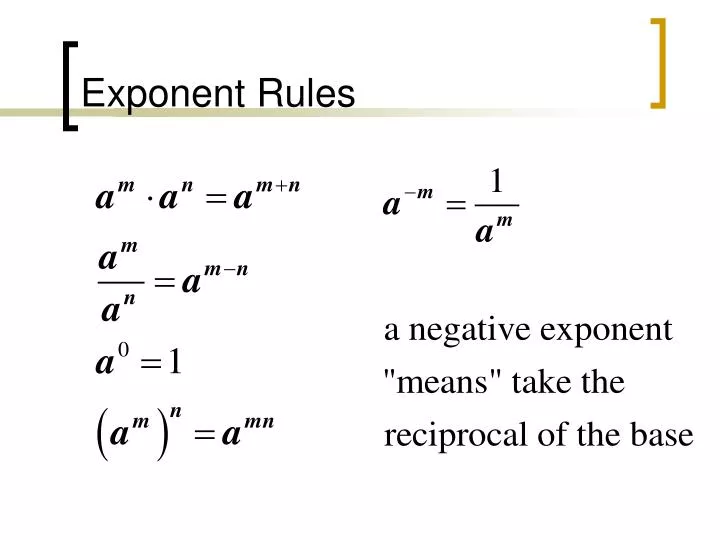
Negative exponent rule reciprocal how to#
Not sure how to convert a decimal number to a fraction? Check out our lesson on converting percentages, decimals, and fractions. 1įrom looking at these tables, you might have already noticed a simpler way to determine the reciprocal of a whole number: Just write a fraction with 1 on top and the original number on the bottom.ĭecimal numbers have reciprocals too! To find the reciprocal of a decimal number, change it to a fraction, then flip the fraction. 5yĪnd because reciprocal means opposite, the reciprocal of a reciprocal fraction is a whole number. Notice that the reciprocal of a number that's already a fraction is just a flipped fraction.
So the reciprocal of 6 is 1/6 because 6 = 6/1 and 1/6 is the inverse of 6/1.īelow, you can see more reciprocals. In other words, the reciprocal has the original fraction's bottom number-or denominator-on top and the top number-or numerator-on the bottom. The reciprocal of a number is this fraction flipped upside down. For example, 6 can also be written as 6/1. To understand the reciprocal, you must first understand that every whole number can be written as a fraction equal to that number divided by 1. It's called the multiplicative inverse, but it's more commonly called a reciprocal. The second type of opposite number has to do with multiplication and division. Reciprocals and the multiplicative inverse Using the additive inverse works for cancelling out because a number added to its inverse always equals 0. In this expression, we're cancelling out -8 by adding its opposite: 8. (If you're not familiar with cancelling out, check out our lesson on simplifying expressions.) When you cancel out a number, you're eliminating it from one side of an equation by performing an inverse action on that number on both sides of the equation. The main time you'll use the additive inverse in algebra is when you cancel out numbers in an expression. For negative numbers or variables, like -5 or -x: Remove the negative sign: -10 → 10.For positive numbers or variables, like 5 or x: Add a negative sign (-) to the left of the number: 5 → -5.If you've never worked with positive and negative numbers, you might want to review our lesson on negative numbers. No matter how large or small a number is, adding it and its inverse will equal 0 every time. In fact, any number you can come up with has an additive inverse. Inverse is just another word for opposite, and additive refers to the fact that when you add these opposite numbers together, they always equal 0. This type of opposite is also called the additive inverse. On a number line, 4 and -4 are both the same distance from 0, but they're on opposite sides. For example, the opposite of 4 is -4, or negative four. The first type of opposite is the one you might be most familiar with: positive numbers and negative numbers. Finding a number's opposites is actually pretty straightforward. Don't be intimidated by these technical-sounding names, though. In fact, every number has two opposites: the additive inverse and the reciprocal-or multiplicative inverse. en/algebra-topics/negative-numbers/content/ Opposite numbersĮvery number has an opposite. Lesson 4: Reciprocals and Inverse Numbers


 0 kommentar(er)
0 kommentar(er)
